|
|
|
A group ![]() of Subsets of
of Subsets of ![]() whose Union contains the given set
whose Union contains the given set ![]() (
(
![]() ) is
called a cover (or a Covering). A Minimal Cover is a cover for which removal of one member destroys
the covering property. There are various types of specialized covers, including proper covers, antichain covers, minimal
covers,
) is
called a cover (or a Covering). A Minimal Cover is a cover for which removal of one member destroys
the covering property. There are various types of specialized covers, including proper covers, antichain covers, minimal
covers, ![]() -covers, and
-covers, and ![]() -covers. The number of possible covers for a set of
-covers. The number of possible covers for a set of ![]() elements is
elements is
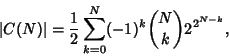
![$\displaystyle \left[{{1\over 2} \sum_{k=0}^N (-1)^k{N\choose k}2^{2^{N-k}}}\right]-{2^{2^N}\over 4},$](c3_697.gif) |
See also Minimal Cover
References
Eppstein, D. ``Covering and Packing.''
http://www.ics.uci.edu/~eppstein/junkyard/cover.html.
Macula, A. J. ``Covers of a Finite Set.'' Math. Mag. 67, 141-144, 1994.
Sloane, N. J. A. Sequences
A003465/M4024
and A007537/M5287
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.