|
|
|
The sum of those terms of the Binomial Series of ![]() for which the number of successes
for which the number of successes ![]() falls between
falls between ![]() and
and ![]() is approximately
is approximately
| (1) |
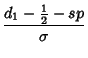 |
(2) | ||
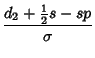 |
(3) | ||
| (4) |
![\begin{displaymath}
Q={1\over\sqrt{2\pi}}\int_{t_1}^{t_2}e^{-t^2/2}\,dt+{q-p\ove...
...t[{(1-t^2){1\over 2\pi} e^{-t^2/2}}\right]^{t_2}_{t_1}+\Omega,
\end{displaymath}](d1_185.gif) |
(5) |
| (6) |
A Corollary states that the probability that ![]() successes in
successes in ![]() trials will differ
from the expected value
trials will differ
from the expected value ![]() by more than
by more than ![]() is
is
| (7) |
| (8) |
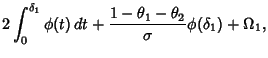 |
(9) |
| (10) | |||
| (11) | |||
| (12) |
| (13) |
References
Uspensky, J. V. Introduction to Mathematical Probability. New York: McGraw-Hill, 1937.
|
|
|
© 1996-9 Eric W. Weisstein