|
|
|
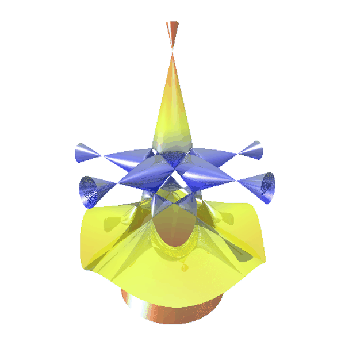
A Quintic Surface having the maximum possible number of Ordinary Double Points (31), which was constructed by W. Barth in 1994 (Endraß). The implicit equation of the surface is
|
|
|
|
|
|
|
|
(1) |
| (2) |
| (3) |
| (4) | |||
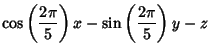 |
(5) | ||
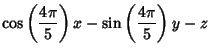 |
(6) | ||
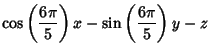 |
(7) | ||
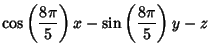 |
(8) |
| (9) |
| (10) | |||
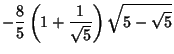 |
(11) | ||
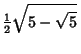 |
(12) |
The dervish is invariant under the Group ![]() and contains exactly 15 lines. Five of these are the intersection of
the surface with a
and contains exactly 15 lines. Five of these are the intersection of
the surface with a ![]() -invariant cone containing 16 nodes, five are the intersection of the surface with a
-invariant cone containing 16 nodes, five are the intersection of the surface with a ![]() -invariant
plane containing 10 nodes, and the last five are the intersection of the surface with a second
-invariant
plane containing 10 nodes, and the last five are the intersection of the surface with a second ![]() -invariant plane
containing no nodes (Endraß).
-invariant plane
containing no nodes (Endraß).
References
Endraß, S. ``Togliatti Surfaces.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Etogliatti.shtml.
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, 4/1995.
Endraß, S. Symmetrische Fläche mit vielen gewöhnlichen Doppelpunkten. Ph.D. thesis. Erlangen, Germany, 1996.
Nordstrand, T. ``Dervish.''
http://www.uib.no/people/nfytn/dervtxt.htm.
|
|
|
© 1996-9 Eric W. Weisstein