|
|
|
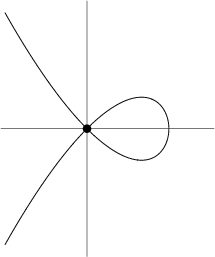
A Rational Double Point of Conic Double Point type, known as ``![]() .'' An ordinary Double Point
is called a Node. The above plot shows the curve
.'' An ordinary Double Point
is called a Node. The above plot shows the curve ![]() , which has an
ordinary double point at the Origin.
, which has an
ordinary double point at the Origin.
A surface in complex 3-space admits at most finitely many ordinary double points. The maximum possible number of
ordinary double points ![]() for a surface of degree
for a surface of degree ![]() , 2, ..., are 0, 1, 4, 16, 31, 65,
, 2, ..., are 0, 1, 4, 16, 31, 65,
![]() ,
,
![]() ,
,
![]() ,
,
![]() ,
,
![]() ,
,
![]() ... (Sloane's A046001; Chmutov 1992, Endraß 1995). The fact that
... (Sloane's A046001; Chmutov 1992, Endraß 1995). The fact that ![]() was proved by
Beauville (1980), and
was proved by
Beauville (1980), and ![]() was proved by Jaffe and Ruberman (1994). For
was proved by Jaffe and Ruberman (1994). For ![]() , the following inequality
holds:
, the following inequality
holds:
| Surface | ||
| 3 | 4 | Cayley Cubic |
| 4 | 16 | Kummer Surface |
| 5 | 31 | Dervish |
| 6 | 65 | Barth Sextic |
| 8 | 168 | Endraß Octic |
| 10 | 345 | Barth Decic |
See also Algebraic Surface, Barth Decic, Barth Sextic, Cayley Cubic, Cusp, Dervish, Endraß Octic, Kummer Surface, Rational Double Point
References
Basset, A. B. ``The Maximum Number of Double Points on a Surface.'' Nature 73, 246, 1906.
Beauville, A. ``Sur le nombre maximum de points doubles d'une surface dans
Chmutov, S. V. ``Examples of Projective Surfaces with Many Singularities.'' J. Algebraic Geom. 1, 191-196, 1992.
Endraß, S. ``Surfaces with Many Ordinary Nodes.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Eflaechen.shtml.
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, Apr. 1995.
Endraß, S. Symmetrische Fläche mit vielen gewöhnlichen Doppelpunkten. Ph.D. thesis. Erlangen, Germany, 1996.
Fischer, G. (Ed.). Mathematical Models from the Collections of Universities and Museums.
Braunschweig, Germany: Vieweg, pp. 12-13, 1986.
Jaffe, D. B. and Ruberman, D. ``A Sextic Surface Cannot have 66 Nodes.'' J. Algebraic Geom. 6, 151-168, 1997.
Miyaoka, Y. ``The Maximal Number of Quotient Singularities on Surfaces with Given Numerical Invariants.'' Math. Ann. 268,
159-171, 1984.
Sloane, N. J. A. Sequence
A046001
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
Togliatti, E. G. ``Sulle superficie algebriche col massimo numero di punti doppi.''
Rend. Sem. Mat. Torino 9, 47-59, 1950.
Varchenko, A. N. ``On the Semicontinuity of Spectrum and an Upper Bound for the Number of Singular Points on a Projective Hypersurface.''
Dokl. Acad. Nauk SSSR 270, 1309-1312, 1983.
Walker, R. J. Algebraic Curves. New York: Springer-Verlag, pp. 56-57, 1978.
![]() (
(![]() ).''
Journées de géométrie algébrique d'Angers (1979). Sijthoff & Noordhoff, pp. 207-215, 1980.
).''
Journées de géométrie algébrique d'Angers (1979). Sijthoff & Noordhoff, pp. 207-215, 1980.
|
|
|
© 1996-9 Eric W. Weisstein