|
|
|
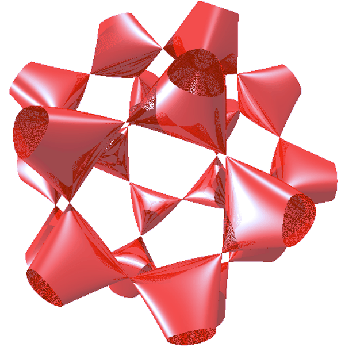
The Barth-sextic is a Sextic Surface in complex three-dimensional projective space having the maximum possible number of Ordinary Double Points (65). It is given by the implicit equation
See also Algebraic Surface, Barth Decic, Cayley Cubic, Ordinary Double Point, Sextic Surface
References
Barth, W. ``Two Projective Surfaces with Many Nodes Admitting the Symmetries of the Icosahedron.'' J. Alg. Geom. 5, 173-186,
1996.
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, 4/1995.
Endraß, S. ``Barth's Sextic.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Ebarthsextic.shtml.
Nordstrand, T. ``Barth Sextic.''
http://www.uib.no/people/nfytn/sexttxt.htm.