|
|
|
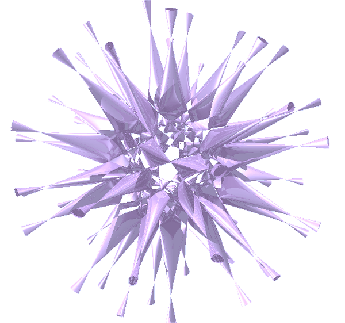
The Barth decic is a Decic Surface in complex three-dimensional projective space having the maximum possible number of Ordinary Double Points (345). It is given by the implicit equation
|
|
|
|
|
See also Algebraic Surface, Barth Sextic, Decic Surface, Ordinary Double Point
References
Barth, W. ``Two Projective Surfaces with Many Nodes Admitting the Symmetries of the Icosahedron.'' J. Alg. Geom. 5, 173-186, 1996.
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, 4/1995.
Endraß, S. ``Barth's Decic.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Ebarthdecic.shtml.
Nordstrand, T. ``Batch Decic.''
http://www.uib.no/people/nfytn/bdectxt.htm.