|
|
|
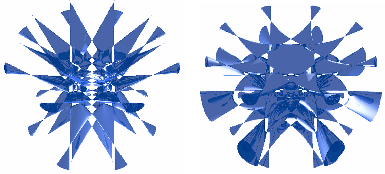
Endraß surfaces are a pair of Octic Surfaces which have 168 Ordinary Double Points. This is the maximum number known to exist for an Octic Surface, although the rigorous upper
bound is 174. The equations of the surfaces ![]() are
are
|
|
|
|
|
|
|
|
See also Octic Surface
References
Endraß, S. ``Octics with 168 Nodes.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Eendrassoctic.shtml.
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, 4/1995.
Endraß, S. ``A Proctive Surface of Degree Eight with 168 Nodes.'' J. Algebraic Geom. 6, 325-334, 1997.