|
|
|
A special case of the Polylogarithm
![]() for
for ![]() . It is denoted
. It is denoted
![]() , or sometimes
, or sometimes ![]() , and is
defined by the sum
, and is
defined by the sum
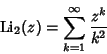
See also Abel's Functional Equation, Polylogarithm, Spence's Integral
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Dilogarithm.'' §27.7 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 1004-1005, 1972.