where 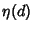 is the Fundamental Unit and
is the Fundamental Unit and 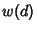 is the number of substitutions which leave the binary quadratic
form unchanged
is the number of substitutions which leave the binary quadratic
form unchanged
See also Class Number, Dirichlet L-Series
References
 Weisstein, E. W. ``Class Numbers.'' Mathematica notebook ClassNumbers.m.
Weisstein, E. W. ``Class Numbers.'' Mathematica notebook ClassNumbers.m.
© 1996-9 Eric W. Weisstein
1999-05-24
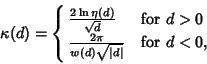
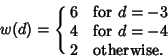
![]() Weisstein, E. W. ``Class Numbers.'' Mathematica notebook ClassNumbers.m.
Weisstein, E. W. ``Class Numbers.'' Mathematica notebook ClassNumbers.m.