The
 function is defined by the integral
function is defined by the integral
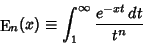 |
(1) |
and is given by the Mathematica
 (Wolfram Research, Champaign, IL) function ExpIntegralE[n,x].
Defining
(Wolfram Research, Champaign, IL) function ExpIntegralE[n,x].
Defining
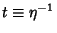 so that
so that
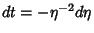 ,
,
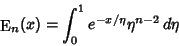 |
(2) |
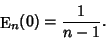 |
(3) |
The function satisfies the Recurrence Relations
 |
(4) |
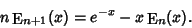 |
(5) |
Equation (4) can be derived from
and (5) using integrating by parts, letting
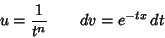 |
(8) |
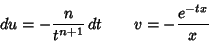 |
(9) |
gives
Solving (10) for
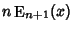 then gives (5). An asymptotic expansion gives
then gives (5). An asymptotic expansion gives
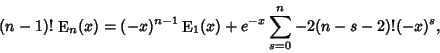 |
(11) |
so
![\begin{displaymath}
\mathop{\rm E}\nolimits_n(x) = {e^{-x}\over x} \left[{1 - {n\over x} + {n(n+1)\over x^2} + \ldots}\right].
\end{displaymath}](e_70.gif) |
(12) |
The special case 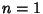 gives
gives
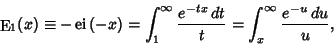 |
(13) |
where
 is the Exponential Integral, which is also equal to
is the Exponential Integral, which is also equal to
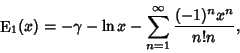 |
(14) |
where 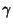 is the Euler-Mascheroni Constant.
is the Euler-Mascheroni Constant.
where
 and
and
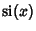 are the Cosine Integral and Sine Integral.
are the Cosine Integral and Sine Integral.
See also Cosine Integral, Et-Function, Exponential Integral, Gompertz Constant,
Sine Integral
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Exponential Integral and Related Functions.'' Ch. 5 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 227-233, 1972.
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. ``Exponential Integrals.'' §6.3 in
Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England:
Cambridge University Press, pp. 215-219, 1992.
Spanier, J. and Oldham, K. B. ``The Exponential Integral Ei(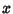 ) and Related Functions.''
Ch. 37 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 351-360, 1987.
) and Related Functions.''
Ch. 37 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 351-360, 1987.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() function is defined by the integral
function is defined by the integral
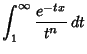
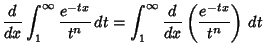
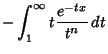
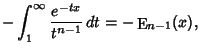
![$\displaystyle \int_1^\infty u\,dv = [uv]_1^\infty-\int_1^\infty v\,du$](e_64.gif)
![$\displaystyle \left[{-{e^{-tx}\over xt^n}}\right]_{t=1}^\infty-{n\over x}\int_1^\infty {e^{-tx}\over t^{n+1}}\,dt$](e_65.gif)
![$\displaystyle \left[{0-\left({-{e^{-x}\over x}}\right)}\right]-{n\over x}\int_1^\infty {e^{-tx}\over t^{n+1}}\,dt$](e_66.gif)
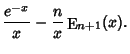
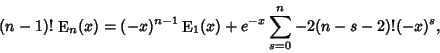
![]() gives
gives
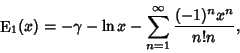
![]() ) and Related Functions.''
Ch. 37 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 351-360, 1987.
) and Related Functions.''
Ch. 37 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 351-360, 1987.