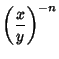The laws governing the combination of Exponents (Powers) are
 |
 |
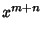 |
(1) |
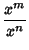 |
 |
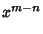 |
(2) |
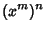 |
 |
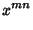 |
(3) |
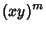 |
 |
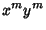 |
(4) |
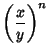 |
 |
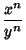 |
(5) |
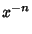 |
 |
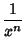 |
(6) |
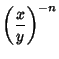 |
 |
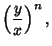 |
(7) |
where quantities in the Denominator are taken to be nonzero. Special cases include
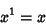 |
(8) |
and
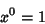 |
(9) |
for 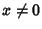 . The definition
. The definition 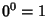 is sometimes used to simplify formulas, but it should be kept in mind
that this equality is a definition and not a fundamental mathematical truth.
is sometimes used to simplify formulas, but it should be kept in mind
that this equality is a definition and not a fundamental mathematical truth.
See also Exponent, Power
© 1996-9 Eric W. Weisstein
1999-05-25