Let 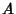 and
and 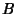 be bilinear forms
be bilinear forms
and suppose that 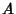 and
and 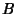 are bounded in
are bounded in ![$[p,p']$](f_271.gif) with bounds
with bounds  and
and 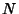 . Then
. Then
where the series
is absolutely convergent, is called the faltung of 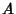 and
and 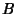 .
. 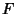 is bounded in
is bounded in ![$[p,p']$](f_271.gif) , and its bound does not exceed
, and its bound does not exceed 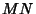 .
.
References
Hardy, G. H.; Littlewood, J. E.; and Pólya, G. Inequalities, 2nd ed. Cambridge,
England: Cambridge University Press, pp. 210-211, 1988.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() and
and ![]() be bilinear forms
be bilinear forms