|
|
|
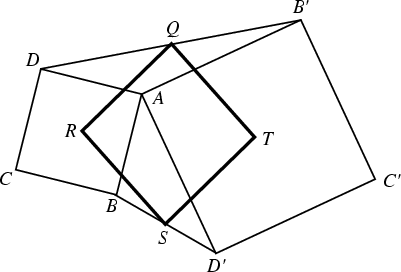
Let the Squares
![]() and
and
![]() share a common Vertex
share a common Vertex ![]() .
The midpoints
.
The midpoints ![]() and
and ![]() of the segments
of the segments ![]() and
and ![]() together with the centers of the original squares
together with the centers of the original squares ![]() and
and ![]() then
form another square
then
form another square
![]() . This theorem is a special case of the Fundamental Theorem of Directly Similar
Figures (Detemple and Harold 1996).
. This theorem is a special case of the Fundamental Theorem of Directly Similar
Figures (Detemple and Harold 1996).
See also Fundamental Theorem of Directly Similar Figures, Square
References
Detemple, D. and Harold, S. ``A Round-Up of Square Problems.'' Math. Mag. 69, 15-27, 1996.
Finsler, P. and Hadwiger, H. ``Einige Relationen im Dreieck.'' Comment. Helv. 10, 316-326, 1937.
Fisher, J. C.; Ruoff, D.; and Shileto, J. ``Polygons and Polynomials.'' In The Geometric Vein: The Coxeter Festschrift.
New York: Springer-Verlag, 321-333, 1981.