|
|
|
The Fourier Transform of a Gaussian Function
![]() is given by
is given by
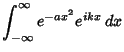 |
|||
![$\displaystyle \int_{-\infty}^\infty e^{-ax^2}[\cos (kx)+i\sin (kx)]\,dx$](f_1878.gif) |
|||
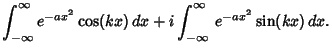 |
See also Gaussian Function
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, 1972.