|
|
|
The Reciprocal of the Arithmetic-Geometric Mean of 1 and ![]() ,
,
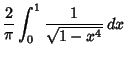 |
(1) | ||
 |
(2) | ||
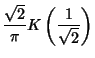 |
|||
| (3) | |||
| (4) |
See also Arithmetic-Geometric Mean, Gauss-Kuzmin-Wirsing Constant
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/gauss/gauss.html