|
|
|
A number given by the Generating Function
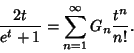
See also Bernoulli Number, Euler Polynomial
References
Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions, rev. enl. ed.
Dordrecht, Netherlands: Reidel, p. 49, 1974.
Kreweras, G. ``An Additive Generation for the Genocchi Numbers and Two of its Enumerative Meanings.'' Bull. Inst. Combin. Appl.
20, 99-103, 1997.
Kreweras, G. ``Sur les permutations comptées par les nombres de Genocchi de 1-ière et 2-ième espèce.''
Europ. J. Comb. 18, 49-58, 1997.
Sloane, N. J. A. Sequence
A001469/M3041
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.