|
|
|
Equivalent to a 2-D Fourier Transform with a radially symmetric Kernel, and also
called the Fourier-Bessel Transform.
| (1) |
| (2) | |||
| (3) |
| (4) | |||
| (5) | |||
| (6) |
| (7) | |||
| (8) | |||
| (9) |
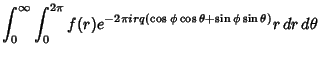 |
|||
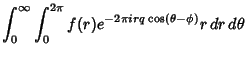 |
|||
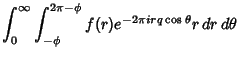 |
|||
 |
|||
![$\displaystyle \int_0^\infty f(r) \left[{\int_0^{2\pi} e^{-2\pi irq\cos \theta }\,d\theta }\right]r\,dr$](h_288.gif) |
|||
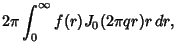 |
(10) |
| (11) |
| (12) |
See also Bessel Function of the First Kind, Fourier Transform, Laplace Transform
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, p. 795, 1985.
Bracewell, R. The Fourier Transform and Its Applications. New York: McGraw-Hill, pp. 244-250, 1965.
|
|
|
© 1996-9 Eric W. Weisstein