In Conical Coordinates, Laplace's Equation can be written
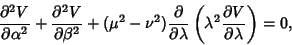 |
(1) |
where
(Byerly 1959). Letting
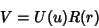 |
(4) |
breaks (1) into the two equations,
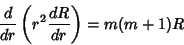 |
(5) |
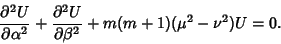 |
(6) |
Solving these gives
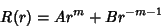 |
(7) |
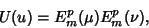 |
(8) |
where 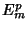 are Ellipsoidal Harmonics. The regular solution is therefore
are Ellipsoidal Harmonics. The regular solution is therefore
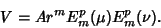 |
(9) |
However, because of the cylindrical symmetry, the solution
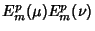 is an
is an 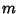 th degree Spherical
Harmonic.
th degree Spherical
Harmonic.
References
Arfken, G. ``Conical Coordinates
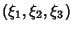 .'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.
.'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.
Byerly, W. E. An Elementary Treatise on Fourier's Series, and Spherical, Cylindrical, and Ellipsoidal Harmonics,
with Applications to Problems in Mathematical Physics. New York: Dover, p. 263, 1959.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 514 and 659, 1953.
© 1996-9 Eric W. Weisstein
1999-05-25
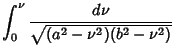
![]() .'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.
.'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.