|
|
|
Arfken (1970) and Morse and Feshbach (1953) use slightly different definitions of these coordinates.
The system used in Mathematica
![]() (Wolfram Research, Inc., Champaign, Illinois) is
(Wolfram Research, Inc., Champaign, Illinois) is
| (1) | |||
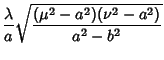 |
(2) | ||
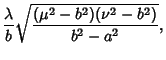 |
(3) |
| (4) |
| (5) |
| (6) |
| (7) | |||
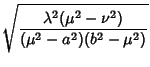 |
(8) | ||
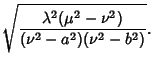 |
(9) |
|
|
|
|
|
(10) |
See also Helmholtz Differential Equation--Conical Coordinates
References
Arfken, G. ``Conical Coordinates (
Byerly, W. E. An Elementary Treatise on Fourier's Series, and Spherical, Cylindrical, and Ellipsoidal Harmonics,
with Applications to Problems in Mathematical Physics. New York: Dover, p. 263, 1959.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, p. 659, 1953.
Spence, R. D. ``Angular Momentum in Sphero-Conal Coordinates.'' Amer. J. Phys. 27, 329-335, 1959.
![]() ,
, ![]() ,
, ![]() ).'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.
).'' §2.16 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 118-119, 1970.
|
|
|
© 1996-9 Eric W. Weisstein