|
|
|
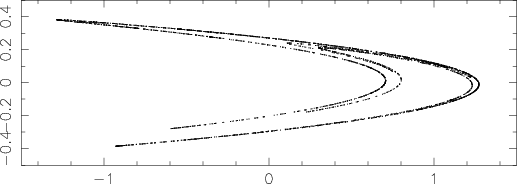
A quadratic 2-D Map given by the equations
| (1) | |||
| (2) |
| (3) | |||
| (4) |
See also Bogdanov Map, Lozi Map, Quadratic Map
References
Dickau, R. M. ``The Hénon Attractor.''
http://forum.swarthmore.edu/advanced/robertd/henon.html.
Gleick, J. Chaos: Making a New Science. New York: Penguin Books, pp. 144-153, 1988.
Grassberger, P. and Procaccia, I. ``Measuring the Strangeness of Strange Attractors.''
Physica D 9, 189-208, 1983.
Hitzl, D. H. and Zele, F. ``An Exploration of the Hénon Quadratic Map.'' Physica D 14, 305-326, 1985.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press,
pp. 128-133, 1991.
Peitgen, H.-O. and Saupe, D. (Eds.). ``A Chaotic Set in the Plane.'' §3.2.2 in
The Science of Fractal Images. New York: Springer-Verlag, pp. 146-148, 1988.
Russell, D. A.; Hanson, J. D.; and Ott, E. ``Dimension of Strange Attractors.'' Phys. Rev. Let. 45, 1175-1178, 1980.