|
|
|
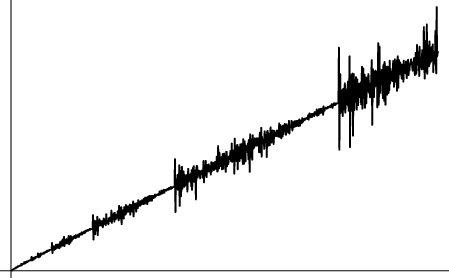
The Integer Sequence given by
See also Hofstadter-Conway $10,000 Sequence, Mallow's Sequence
References
Conolly, B. W. ``Meta-Fibonacci Sequences.'' In Fibonacci and Lucas Numbers, and the Golden Section
(Ed. S. Vajda). New York: Halstead Press, pp. 127-138, 1989.
Guy, R. ``Some Suspiciously Simple Sequences.'' Amer. Math. Monthly 93, 186-191, 1986.
Hofstadter, D. R. Gödel, Escher Bach: An Eternal Golden Braid. New York: Vintage Books, pp. 137-138, 1980.
Pickover, C. A. ``The Crying of Fractal Batrachion 1,489.'' Ch. 25 in Keys to Infinity. New York:
W. H. Freeman, pp. 183-191, 1995.
Sloane, N. J. A. Sequence
A005185/M0438
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.