|
|
|
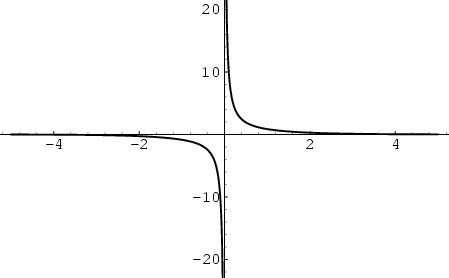
The hyperbolic cosecant is defined as
See also Bernoulli Number, Bipolar Coordinates, Bipolar Cylindrical Coordinates, Cosecant, Helmholtz Differential Equation--Toroidal Coordinates, Hyperbolic Sine, Poinsot's Spirals, Surface of Revolution, Toroidal Function
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Hyperbolic Functions.'' §4.5 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 83-86, 1972.
Spanier, J. and Oldham, K. B. ``The Hyperbolic Secant
![]() and Cosecant
and Cosecant
![]() Functions.''
Ch. 29 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 273-278, 1987.
Functions.''
Ch. 29 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 273-278, 1987.