|
|
|
By analogy with the Lemniscate Functions, hyperbolic lemniscate functions can also be
defined
| (1) |
| (2) |
| (3) |
| (4) |
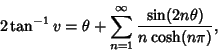 |
(5) |
![\begin{displaymath}
{\textstyle{1\over 8}}\pi-{\textstyle{1\over 2}}\tan^{-1}(v^...
...2n+1)\theta]\over(2n+1)\cosh[{\textstyle{1\over 2}}(2n+1)\pi]}
\end{displaymath}](h_2109.gif) |
(6) |
![\begin{displaymath}
\ln\left({1+v\over 1-v}\right)=\ln[\tan({\textstyle{1\over 4...
...\infty {(-1)^n\sin[(2n+1)\theta]\over (2n+1)[e^{(2n+1)\pi}-1]}
\end{displaymath}](h_2110.gif) |
(7) |
See also Lemniscate Function
References
Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 255-258, 1994.