|
|
|
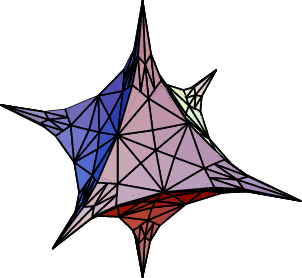
A hyperbolic version of the Euclidean Octahedron, which is a special case of the Astroidal Ellipsoid with
![]() . It is given by the parametric equations
. It is given by the parametric equations
See also Astroidal Ellipsoid, Hyperbolic Cube, Hyperbolic Dodecahedron, Hyperbolic Tetrahedron
References
Gray, A. Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, pp. 305-306, 1993.
Nordstrand, T. ``Astroidal Ellipsoid.''
http://www.uib.no/people/nfytn/asttxt.htm.
![]() Rivin, I. ``Hyperbolic Polyhedron Graphics.''
http://www.mathsource.com/cgi-bin/MathSource/Applications/Graphics/3D/0201-788.
Rivin, I. ``Hyperbolic Polyhedron Graphics.''
http://www.mathsource.com/cgi-bin/MathSource/Applications/Graphics/3D/0201-788.