|
|
|
|
A Platonic Solid (![]() ) with six Vertices, 12 Edges, and eight equivalent Equilateral Triangular faces (
) with six Vertices, 12 Edges, and eight equivalent Equilateral Triangular faces (![]() ), given by
the Schläfli Symbol
), given by
the Schläfli Symbol ![]() . It is also Uniform Polyhedron
. It is also Uniform Polyhedron ![]() with the
Wythoff Symbol
with the
Wythoff Symbol ![]() . Its Dual Polyhedron is the Cube. Like the Cube, it has the
. Its Dual Polyhedron is the Cube. Like the Cube, it has the
![]() Octahedral Group of symmetries. The octahedron can be Stellated to give the
Stella Octangula.
Octahedral Group of symmetries. The octahedron can be Stellated to give the
Stella Octangula.
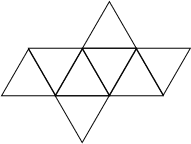
The solid bounded by the two Tetrahedra of the Stella Octangula (left figure) is an octahedron (right figure; Ball and Coxeter 1987).
In one orientation (left figure), the Vertices are given by ![]() ,
, ![]() ,
,
![]() . In another orientation (right figure), the vertices are
. In another orientation (right figure), the vertices are
![]() and
and
![]() . In the
latter, the constituent Triangles are specified by
. In the
latter, the constituent Triangles are specified by
| (1) |
A plane Perpendicular to a ![]() axis of an octahedron cuts the solid in a regular Hexagonal
Cross-Section (Holden 1991, pp. 22-23). Since there are four such axes, there are four possibly
Hexagonal Cross-Sections. Faceted forms are the Cuboctatruncated
Cuboctahedron and Tetrahemihexahedron.
axis of an octahedron cuts the solid in a regular Hexagonal
Cross-Section (Holden 1991, pp. 22-23). Since there are four such axes, there are four possibly
Hexagonal Cross-Sections. Faceted forms are the Cuboctatruncated
Cuboctahedron and Tetrahemihexahedron.
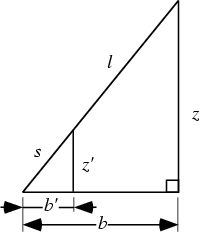
Let an octahedron be length ![]() on a side. The height of the top Vertex from the square
plane is also the Circumradius
on a side. The height of the top Vertex from the square
plane is also the Circumradius
| (2) |
| (3) |
| (4) |
| (5) | |||
| (6) | |||
| (7) |
| (8) |
| (9) | |||
| (10) | |||
| (11) |
| (12) |
| (13) |
The Area of one face is the Area of an Equilateral Triangle
| (14) |
| (15) |
| (16) |
See also Octahedral Graph, Octahedral Group, Octahedron 5-Compound, Stella Octangula, Truncated Octahedron
References
Davie, T. ``The Octahedron.''
http://www.dcs.st-and.ac.uk/~ad/mathrecs/polyhedra/octahedron.html.
Holden, A. Shapes, Space, and Symmetry. New York: Dover, 1991.
|
|
|
© 1996-9 Eric W. Weisstein