Circumradius

The radius of a Triangle's Circumcircle or of a Polyhedron's Circumsphere, denoted $R$. For a Triangle,

$$R={abc\over \sqrt{(a+b+c)(b+c-a)(c+a-b)(a+b-c)}},$$ (1)

where the side lengths of the Triangle are $a$, $b$, and $c$.

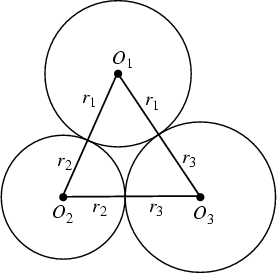
This equation can also be expressed in terms of the Radii of the three mutually tangent Circles centered at the Triangle's Vertices. Relabeling the diagram for the Soddy Circles with Vertices $O_1$, $O_2$, and $O_3$ and the radii $r_1$, $r_2$, and $r_3$, and using

$$a = r_1+r_2$$ (2)

$$b = r_2+r_3$$ (3)

$$c = r_1+r_3$$ (4)

then gives

$$R={(r_1+r_2)(r_1+r_3)(r_2+r_3)\over 4\sqrt{r_1r_2r_3(r_1+r_2+r_3)}}.$$ (5)

If $O$ is the Circumcenter and $M$ is the triangle Centroid, then

$$\overline{OM}^2=R^2-{\textstyle{1\over 9}}(a^2+b^2+c^2).$$ (6)

$$Rr={a_1a_2a_3\over 4s}$$ (7)

$$\cos\alpha_1+\cos\alpha_2+\cos\alpha_3=1+{r\over R}$$ (8)

$$r=2R\cos\alpha_1\cos\alpha_2\cos\alpha_3$$ (9)

$${a_1}^2+{a_2}^2+{a_3}^2=4rR+8R^2$$ (10)

(Johnson 1929, pp. 189-191). Let $d$ be the distance between Inradius $r$ and circumradius $R$, $d=\overline{rR}$. Then

$$R^2-d^2=2Rr$$ (11)

$${1\over R-d}+{1\over R+d}={1\over r}$$ (12)

(Mackay 1886-87). These and many other identities are given in Johnson (1929, pp. 186-190).

For an Archimedean Solid, expressing the circumradius in terms of the Inradius $r$ and Midradius $\rho$ gives

$$R = {\textstyle{1\over 2}}(r+\sqrt{r^2+a^2}\,)$$ (13)

$$= \sqrt{\rho^2+{\textstyle{1\over 4}}a^2}$$ (14)

for an Archimedean Solid.

See also Carnot's Theorem, Circumcircle, Circumsphere

References

Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, 1929.

Mackay, J. S. ``Historical Notes on a Geometrical Theorem and its Developments [18th Century].'' Proc. Edinburgh Math. Soc. 5, 62-78, 1886-1887.