|
|
|
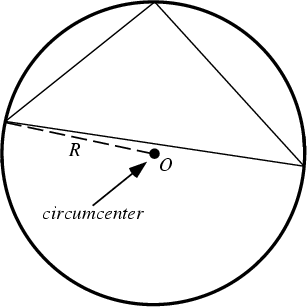
The center ![]() of a Triangle's Circumcircle. It can be found as the intersection of the
Perpendicular Bisectors. If the Triangle is Acute,
the circumcenter is in the interior of the Triangle. In a Right Triangle, the circumcenter is the
Midpoint of the Hypotenuse.
of a Triangle's Circumcircle. It can be found as the intersection of the
Perpendicular Bisectors. If the Triangle is Acute,
the circumcenter is in the interior of the Triangle. In a Right Triangle, the circumcenter is the
Midpoint of the Hypotenuse.
| (1) |
| (2) |
| (3) |
| (4) |
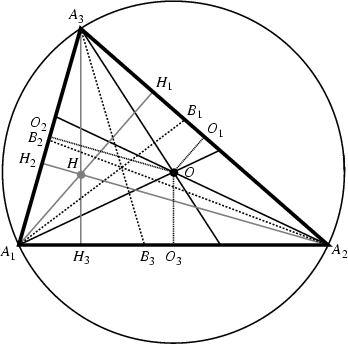
The circumcenter ![]() and Orthocenter
and Orthocenter ![]() are Isogonal Conjugates.
are Isogonal Conjugates.
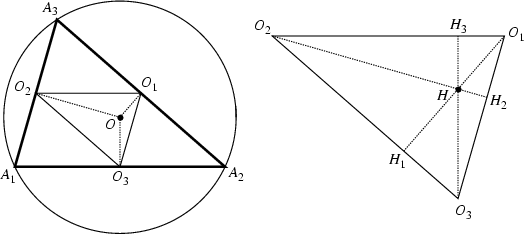
The Orthocenter ![]() of the Pedal Triangle
of the Pedal Triangle
![]() formed by the Circumcenter
formed by the Circumcenter ![]() concurs with
the circumcenter
concurs with
the circumcenter ![]() itself, as illustrated above. The circumcenter also lies on the Euler Line.
itself, as illustrated above. The circumcenter also lies on the Euler Line.
See also Brocard Diameter, Carnot's Theorem, Centroid (Triangle), Circle, Euler Line, Incenter, Orthocenter
References
Carr, G. S. Formulas and Theorems in Pure Mathematics, 2nd ed. New York: Chelsea, p. 623, 1970.
Dixon, R. Mathographics. New York: Dover, p. 55, 1991.
Eppstein, D. ``Circumcenters of Triangles.''
http://www.ics.uci.edu/~eppstein/junkyard/circumcenter.html.
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, 1929.
Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.
Kimberling, C. ``Circumcenter.''
http://cedar.evansville.edu/~ck6/tcenters/class/ccenter.html.
|
|
|
© 1996-9 Eric W. Weisstein