|
|
|
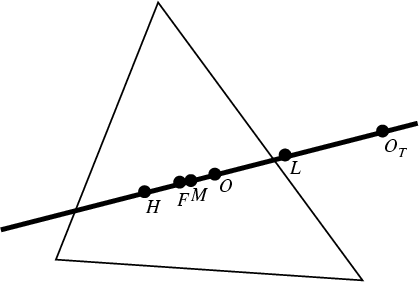
The line on which the Orthocenter ![]() , Centroid
, Centroid ![]() , Circumcenter
, Circumcenter
![]() , de Longchamps Point
, de Longchamps Point ![]() , Nine-Point Center
, Nine-Point Center ![]() , and the Tangential Triangle Circumcircle
, and the Tangential Triangle Circumcircle
![]() of a Triangle lie. The Incenter lies on the Euler line only if the Triangle is an
Isosceles Triangle. The Euler line consists of all points with Trilinear Coordinates
of a Triangle lie. The Incenter lies on the Euler line only if the Triangle is an
Isosceles Triangle. The Euler line consists of all points with Trilinear Coordinates
![]() which satisfy
which satisfy
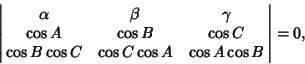 |
(1) |
|
|
|
|
|
(2) |
| (3) |
| (4) |
| Center | |
| point at infinity | |
| de Longchamps Point |
|
| 0 | Circumcenter |
| 1 | Centroid |
| 2 | Nine-Point Center |
| Orthocenter |
The Orthocenter is twice as far from the Centroid as is the
Circumcenter. The Circumcenter ![]() , Nine-Point Center
, Nine-Point Center ![]() , Centroid
, Centroid
![]() , and Orthocenter
, and Orthocenter ![]() form a Harmonic Range.
form a Harmonic Range.
The Euler line intersects the Soddy Line in the de Longchamps Point, and the Gergonne Line in the Evans Point. The Isotomic Conjugate of the Euler line is called Jerabek's Hyperbola (Casey 1893, Vandeghen 1965).
See also Centroid (Triangle), Circumcenter, Evans Point, Gergonne Line, Jerabek's Hyperbola, de Longchamps Point, Nine-Point Center, Orthocenter, Soddy Line, Tangential Triangle
References
Casey, J. A Treatise on the Analytical Geometry of the Point, Line, Circle, and Conic Sections, Containing
an Account of Its Most Recent Extensions with Numerous Examples, 2nd rev. enl. ed. Dublin: Hodges, Figgis, & Co., 1893.
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 18-20, 1967.
Dörrie, H. ``Euler's Straight Line.'' §27 in 100 Great Problems of Elementary Mathematics: Their History and Solutions.
New York: Dover, pp. 141-142, 1965.
Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 117-119, 1990.
Oldknow, A. ``The Euler-Gergonne-Soddy Triangle of a Triangle.'' Amer. Math. Monthly 103, 319-329, 1996.
Vandeghen, A. ``Some Remarks on the Isogonal and Cevian Transforms. Alignments of Remarkable Points of a Triangle.''
Amer. Math. Monthly 72, 1091-1094, 1965.
|
|
|
© 1996-9 Eric W. Weisstein