|
|
|
A six-sided Polygon. In proposition IV.15, Euclid ![]() showed how to inscribe a regular hexagon in a
Circle. The Inradius
showed how to inscribe a regular hexagon in a
Circle. The Inradius ![]() , Circumradius
, Circumradius ![]() , and Area
, and Area ![]() can be computed directly from the formulas
for a general regular Polygon with side length
can be computed directly from the formulas
for a general regular Polygon with side length ![]() and
and ![]() sides,
sides,
| (1) | |||
| (2) | |||
| (3) |
| (4) |
| (5) |
A Plane Perpendicular to a ![]() axis of a Cube, Dodecahedron, or Icosahedron cuts the
solid in a regular Hexagonal Cross-Section (Holden 1991, pp. 22-23 and 27). For the Cube,
the Plane passes through the Midpoints of opposite sides (Steinhaus 1983, p. 170; Cundy and
Rollett 1989, p. 157; Holden 1991, pp. 22-23). Since there are four such axes for the Cube and Octahedron,
there are four possible hexagonal cross-sections.
axis of a Cube, Dodecahedron, or Icosahedron cuts the
solid in a regular Hexagonal Cross-Section (Holden 1991, pp. 22-23 and 27). For the Cube,
the Plane passes through the Midpoints of opposite sides (Steinhaus 1983, p. 170; Cundy and
Rollett 1989, p. 157; Holden 1991, pp. 22-23). Since there are four such axes for the Cube and Octahedron,
there are four possible hexagonal cross-sections.
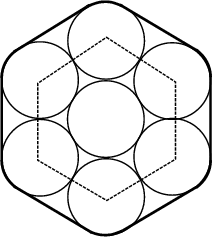
Take seven Circles and close-pack them together in a hexagonal arrangement. The Perimeter obtained
by wrapping a band around the Circle then consists of six straight segments of length ![]() (where
(where ![]() is
the Diameter) and 6 arcs with total length
is
the Diameter) and 6 arcs with total length ![]() of a Circle. The Perimeter is therefore
of a Circle. The Perimeter is therefore
| (6) |
See also Cube, Cyclic Hexagon, Dissection, Dodecahedron, Graham's Biggest Little Hexagon, Hexagon Polyiamond, Hexagram, Magic Hexagon, Octahedron, Pappus's Hexagon Theorem, Pascal's Theorem, Talisman Hexagon
References
Cundy, H. and Rollett, A. ``Hexagonal Section of a Cube.'' §3.15.1 in Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., p. 157, 1989.
Dixon, R. Mathographics. New York: Dover, p. 16, 1991.
Holden, A. Shapes, Space, and Symmetry. New York: Dover, 1991.
Pappas, T. ``Hexagons in Nature.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 74-75, 1989.
Steinhaus, H. Mathematical Snapshots, 3rd American ed. New York: Oxford University Press, 1983.
|
|
|
© 1996-9 Eric W. Weisstein