|
|
|
A Platonic Solid (![]() ) with 12 Vertices, 30 Edges, and 20 equivalent
Equilateral Triangle faces
) with 12 Vertices, 30 Edges, and 20 equivalent
Equilateral Triangle faces ![]() . It is described by the Schläfli Symbol
. It is described by the Schläfli Symbol
![]() . It is also Uniform Polyhedron
. It is also Uniform Polyhedron ![]() and has Wythoff Symbol
and has Wythoff Symbol ![]() . The icosahedron has
the Icosahedral Group
. The icosahedron has
the Icosahedral Group ![]() of symmetries.
of symmetries.
A plane Perpendicular to a ![]() axis of an icosahedron cuts the solid in a regular Decagonal
Cross-Section (Holden 1991, pp. 24-25).
axis of an icosahedron cuts the solid in a regular Decagonal
Cross-Section (Holden 1991, pp. 24-25).
A construction for an icosahedron with side length
![]() places the end vertices at
places the end vertices at ![]() )
and the central vertices around two staggered Circles of Radii
)
and the central vertices around two staggered Circles of Radii
![]() and
heights
and
heights
![]() , giving coordinates
, giving coordinates
| (1) |
| (2) | |||
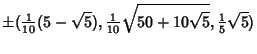 |
(3) | ||
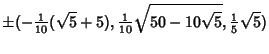 |
(4) | ||
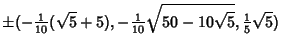 |
(5) | ||
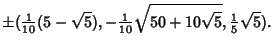 |
(6) |
The Dual Polyhedron of the icosahedron is the Dodecahedron. There are 59 distinct icosahedra when each Triangle is colored differently (Coxeter 1969).

To derive the Volume of an icosahedron having edge length ![]() , consider the orientation so that two
Vertices are oriented on top and bottom. The vertical distance between the top and bottom
Pentagonal Dipyramids is then given by
, consider the orientation so that two
Vertices are oriented on top and bottom. The vertical distance between the top and bottom
Pentagonal Dipyramids is then given by
| (7) |
| (8) |
| (9) |
| (10) |
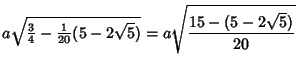 |
|||
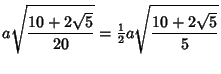 |
|||
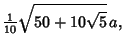 |
(11) |
| (12) |
| (13) |
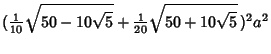 |
|||
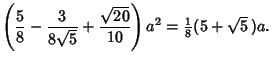 |
(14) |
| (15) |
| (16) |
| (17) |
| (18) |
The Area of one face is the Area of an Equilateral Triangle
| (19) |
| (20) |
| (21) |
See also Augmented Tridiminished Icosahedron, Decagon, Dodecahedron, Great Icosahedron, Icosahedron Stellations, Metabidiminished Icosahedron, Tridiminished Icosahedron, Trigonometry Values Pi/5
References
Coxeter, H. S. M. Introduction to Geometry, 2nd ed. New York: Wiley, 1969.
Davie, T. ``The Icosahedron.''
http://www.dcs.st-and.ac.uk/~ad/mathrecs/polyhedra/icosahedron.html.
Holden, A. Shapes, Space, and Symmetry. New York: Dover, 1991.
Klein, F. Lectures on the Icosahedron. New York: Dover, 1956.
Pappas, T. ``The Icosahedron & the Golden Rectangle.'' The Joy of Mathematics.
San Carlos, CA: Wide World Publ./Tetra, p. 115, 1989.
|
|
|
© 1996-9 Eric W. Weisstein