|
|
|
A joint distribution function is a Distribution Function ![]() in two variables defined by
in two variables defined by
| (1) | |||
| (2) | |||
| (3) |
| (4) |
| (5) |
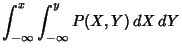 |
(6) |
| (7) |
A multiple distribution function is of the form
| (8) |
See also Distribution Function
References
Grimmett, G. and Stirzaker, D. Probability and Random Processes, 2nd ed. New York: Oxford University Press, 1992.