|
|
|
Let ![]() be a Bessel Function of the First Kind,
be a Bessel Function of the First Kind, ![]() a Neumann Function, and
a Neumann Function, and ![]() the
zeros of
the
zeros of
![]() in order of ascending Real Part. Then for
in order of ascending Real Part. Then for ![]() and
and ![]() ,
,
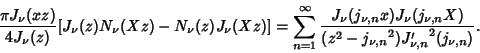
References
Iyanaga, S. and Kawada, Y. (Eds.). Encyclopedic Dictionary of Mathematics. Cambridge, MA: MIT Press,
p. 1474, 1980.