Given a Fermat Difference Equation (a quadratic Diophantine Equation)
with 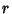 a Quadratic Surd, assign to each solution
a Quadratic Surd, assign to each solution 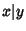 the Lagrange number
the Lagrange number
The product and quotient of two Lagrange numbers are also Lagrange numbers. Furthermore, every Lagrange number is a
Power of the smallest Lagrange number with an integral exponent.
See also Pell Equation
References
Dörrie, H. 100 Great Problems of Elementary Mathematics: Their History and Solutions. New York: Dover,
pp. 94-95, 1965.
© 1996-9 Eric W. Weisstein
1999-05-26