Consider the general system of two first-order Ordinary Differential Equations
Let 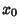 and
and 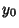 denote Fixed Points with
denote Fixed Points with
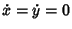 , so
, so
Then expand about 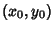 so
so
To first-order, this gives
![\begin{displaymath}
{d\over dt}\left[{\matrix{\delta x\cr \delta y}}\right] = \l...
...\cr}}\right]
\left[{\matrix{\delta x\cr \delta y\cr}}\right],
\end{displaymath}](l2_292.gif) |
(7) |
where the 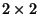 Matrix is called the Stability Matrix.
Matrix is called the Stability Matrix.
In general, given an 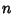 -D Map
-D Map
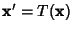 , let
, let 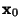 be a Fixed Point, so that
be a Fixed Point, so that
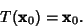 |
(8) |
Expand about the fixed point,
so
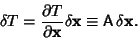 |
(10) |
The map can be transformed into the principal axis frame by finding the Eigenvectors and
Eigenvalues of the Matrix A
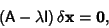 |
(11) |
so the Determinant
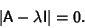 |
(12) |
The mapping is
![\begin{displaymath}
\delta{{\bf x}_{\rm princ}}' = \left[{\matrix{\lambda_1 & \c...
...ots & \ddots & \vdots \cr 0 & \cdots & \lambda_n \cr}}\right].
\end{displaymath}](l2_304.gif) |
(13) |
When iterated a large number of times,
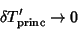 |
(14) |
only if
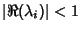 for
for  , ...,
, ..., 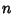 but
but 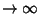 if any
if any 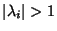 . Analysis of the
Eigenvalues (and Eigenvectors) of A therefore characterizes the type
of Fixed Point. The condition for stability is
. Analysis of the
Eigenvalues (and Eigenvectors) of A therefore characterizes the type
of Fixed Point. The condition for stability is
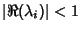 for
for  , ...,
, ..., 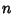 .
.
See also Fixed Point, Stability Matrix
References
Tabor, M. ``Linear Stability Analysis.'' §1.4 in
Chaos and Integrability in Nonlinear Dynamics: An Introduction. New York: Wiley, pp. 20-31, 1989.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() -D Map
-D Map
![]() , let
, let ![]() be a Fixed Point, so that
be a Fixed Point, so that
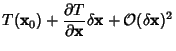
![\begin{displaymath}
\delta{{\bf x}_{\rm princ}}' = \left[{\matrix{\lambda_1 & \c...
...ots & \ddots & \vdots \cr 0 & \cdots & \lambda_n \cr}}\right].
\end{displaymath}](l2_304.gif)