|
|
|
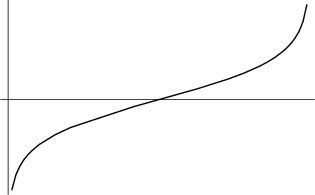
The function
References
Collins, J.; Mancilulli, M.; Hohlfeld, R.; Finch, D.; Sandri, G.; and Shtatland, E. ``A Random Number Generator
Based on the Logit Transform of the Logistic Variable.'' Computers in Physics 6, 630-632, 1992.
Pickover, C. A. Keys to Infinity. New York: Wiley, pp. 244-245, 1995.