The Lorentzian function is given by
Its Full Width at Half Maximum is 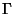 . This function gives the shape of certain types of spectral lines and is the
distribution function in the Cauchy Distribution. The Lorentzian function has Fourier Transform
. This function gives the shape of certain types of spectral lines and is the
distribution function in the Cauchy Distribution. The Lorentzian function has Fourier Transform
See also Damped Exponential Cosine Integral, Fourier Transform--Lorentzian Function
© 1996-9 Eric W. Weisstein
1999-05-25