|
|
|
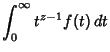 |
|||
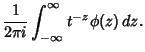 |
See also Strassen Formulas
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, p. 795, 1985.
Bracewell, R. The Fourier Transform and Its Applications. New York: McGraw-Hill, pp. 254-257, 1965.
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 469-471, 1953.