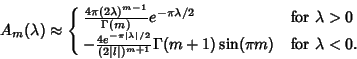where the function
describes the motion along the pendulum  Separatrix. Chirikov (1979) has shown that this integral
has the approximate value
Separatrix. Chirikov (1979) has shown that this integral
has the approximate value
References
Chirikov, B. V. ``A Universal Instability of Many-Dimensional Oscillator Systems.'' Phys. Rep. 52, 264-379, 1979.
© 1996-9 Eric W. Weisstein
1999-05-26