The equation
represents an 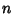 -D Hypersphere
-D Hypersphere 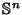 as a quadratic hypersurface in an
as a quadratic hypersurface in an 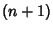 -D real projective space
-D real projective space
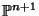 , where
, where 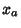 are homogeneous coordinates in
are homogeneous coordinates in 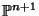 . Then the Group
. Then the Group 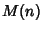 of projective
transformations which leave
of projective
transformations which leave 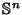 invariant is called the Möbius group.
invariant is called the Möbius group.
References
Iyanaga, S. and Kawada, Y. (Eds.). ``Möbius Geometry.'' §78A in
Encyclopedic Dictionary of Mathematics. Cambridge, MA: MIT Press, pp. 265-266, 1980.
© 1996-9 Eric W. Weisstein
1999-05-26