|
|
|
Given a Sequence
![]() , an
, an ![]() -moving average is a new sequence
-moving average is a new sequence
![]() defined from
the
defined from
the ![]() by taking the Average of subsequences of
by taking the Average of subsequences of ![]() terms,
terms,
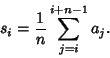
See also Average, Spencer's 15-Point Moving Average
References
Kenney, J. F. and Keeping, E. S. ``Moving Averages.'' §14.2 in Mathematics of Statistics, Pt. 1, 3rd ed.
Princeton, NJ: Van Nostrand, pp. 221-223, 1962.