Consider a Knot as being formed from two Tangles.
The following three operations are called mutations.
- 1. Cut the knot open along four points on each of the four strings coming out of
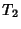 , flipping
, flipping 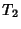 over, and
gluing the strings back together.
over, and
gluing the strings back together.
- 2. Cut the knot open along four points on each of the four strings coming out of
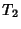 , flipping
, flipping 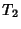 to the
right, and gluing the strings back together.
to the
right, and gluing the strings back together.
- 3. Cut the knot, rotate it by 180°, and reglue. This is equivalent
to performing (1), then (2).
Mutations applied to an alternating Knot projection always yield an Alternating Knot. The mutation of a
Knot is always another Knot (a opposed to a Link).
References
Adams, C. C. The Knot Book: An Elementary Introduction to the Mathematical Theory of Knots.
New York: W. H. Freeman, p. 49, 1994.
© 1996-9 Eric W. Weisstein
1999-05-26