|
|
|
A skewed distribution which is similar to the Binomial Distribution when ![]() (Abramowitz and Stegun 1972, p. 930).
(Abramowitz and Stegun 1972, p. 930).
| (1) |
| (2) | |||
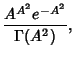 |
(3) |
| (4) |
| (5) |
| (6) | |||
| (7) | |||
| (8) | |||
| (9) |
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, 1972.