A Figurate Number which is given by
where 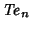 is the
is the 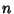 th Tetrahedral Number. The first few pentatope numbers are 1, 5, 15, 35, 70, 126, ...
(Sloane's A000332). The Generating Function for the pentatope numbers is
th Tetrahedral Number. The first few pentatope numbers are 1, 5, 15, 35, 70, 126, ...
(Sloane's A000332). The Generating Function for the pentatope numbers is
See also Figurate Number, Tetrahedral Number
References
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 55-57, 1996.
© 1996-9 Eric W. Weisstein
1999-05-26