|
|
|
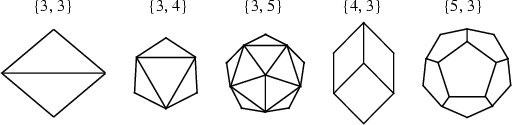
A skew Polygon such that every two consecutive sides (but no three) belong to a face of a regular Polyhedron.
Every finite Polyhedron can be orthogonally projected onto a plane in such a way that one Petrie polygon becomes a
Regular Polygon with the remainder of the projection interior to it. The Petrie polygon of the Polyhedron
![]() has
has ![]() sides, where
sides, where
See also Platonic Solid, Regular Polygon
References
Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 135, 1987.
Coxeter, H. S. M. ``Petrie Polygons.'' §2.6 in Regular Polytopes, 3rd ed. New York: Dover, pp.24-25, 1973.