|
|
|
A two-dimensional array of Integers nonincreasing both left to right and top to bottom which add
up to a given number, i.e.,
![]() and
and
![]() . For example, a planar partition of
22 is given by
. For example, a planar partition of
22 is given by
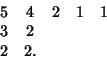
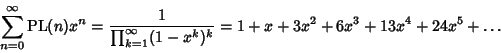
See also Partition, Solid Partition
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. Item 18 in HAKMEM. Cambridge, MA: MIT
Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.
Bender, E. A. and Knuth, D. E. ``Enumeration of Plane Partitions.'' J. Combin. Theory Ser. A. 13,
40-54, 1972.
Knuth, D. E. ``A Note on Solid Partitions.'' Math. Comput. 24, 955-961, 1970.
MacMahon, P. A. ``Memoir on the Theory of the Partitions of Numbers. V: Partitions in Two-Dimensional Space.''
Phil. Trans. Roy. Soc. London Ser. A 211, 75-110, 1912a.
MacMahon, P. A. ``Memoir on the Theory of the Partitions of Numbers. VI: Partitions in Two-Dimensional Space,
to which is Added an Adumbration of the Theory of Partitions in Three-Dimensional Space.''
Phil. Trans. Roy. Soc. London Ser. A 211, 345-373, 1912b.
MacMahon, P. A. Combinatory Analysis, Vol. 2. New York: Chelsea, 1960.
Sloane, N. J. A. Sequence
A000219/M2566
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.