|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Define the function
| (1) |
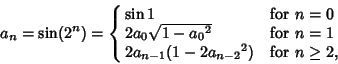 |
(2) |
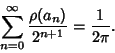 |
(3) |
For
| (4) |
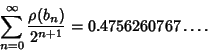 |
(5) |
Letting
| (6) |
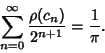 |
(7) |
Plouffe asked if the above processes could be ``inverted.'' He considered
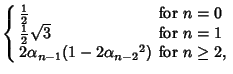 |
(8) |
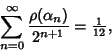 |
(9) |
| (10) |
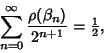 |
(11) |
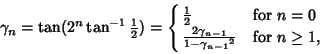 |
(12) |
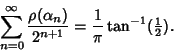 |
(13) |
Borwein and Girgensohn (1995) extended Plouffe's ![]() to arbitrary Real
to arbitrary Real ![]() , showing
that if
, showing
that if
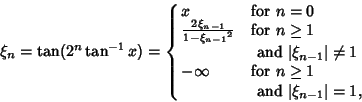 |
(14) |
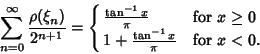 |
(15) |
References
Borwein, J. M. and Girgensohn, R. ``Addition Theorems and Binary Expansions.'' Canad. J. Math. 47, 262-273, 1995.
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/plff/plff.html
Plouffe, S.. ``The Computation of Certain Numbers Using a Ruler and Compass.'' Dec. 12, 1997.
http://www.research.att.com/~njas/sequences/JIS/compass.html.
Sloane, N. J. A. Sequence
A004715
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
|
|
|
© 1996-9 Eric W. Weisstein