|
|
|
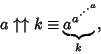
See also Ackermann Function, Fermat Number, Mills' Constant
References
Knuth, D. E. ``Mathematics and Computer Science: Coping with Finiteness. Advances in our Ability to Compute are
Bringing us Substantially Closer to Ultimate Limitations.'' Science 194 1235-1242, 1976.
Vardi, I. Computational Recreations in Mathematica. Reading, MA: Addison-Wesley, pp. 11 and 226-229, 1991.