The total Power of a Triangle is defined by
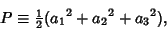 |
(1) |
where 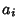 are the side lengths, and the ``partial power'' is defined by
are the side lengths, and the ``partial power'' is defined by
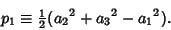 |
(2) |
Then
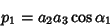 |
(3) |
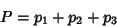 |
(4) |
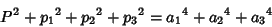 |
(5) |
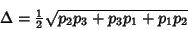 |
(6) |
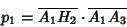 |
(7) |
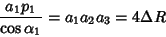 |
(8) |
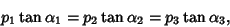 |
(9) |
where 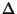 is the Area of the Triangle and
is the Area of the Triangle and 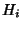 are the Feet of the Altitudes. Finally, if a side of the Triangle and the value of any partial power are given, then the Locus of
the third Vertex is a Circle or straight line.
are the Feet of the Altitudes. Finally, if a side of the Triangle and the value of any partial power are given, then the Locus of
the third Vertex is a Circle or straight line.
See also Altitude, Foot, Triangle
References
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, pp. 260-261, 1929.
© 1996-9 Eric W. Weisstein
1999-05-26