|
|
|
The regularized gamma functions are defined by
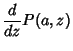 |
|||
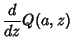 |
See also Gamma Function, Regularized Beta Function
References
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T.
Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England:
Cambridge University Press, pp. 160-161, 1992.