|
|
|
For the Helmholtz Differential Equation to be Separable in a coordinate system, the Scale Factors ![]() in the Laplacian
in the Laplacian
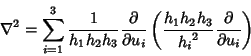 |
(1) |
| (2) |
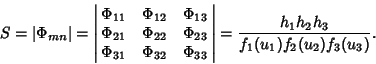 |
(3) |
See also Helmholtz Differential Equation, Laplace's Equation, Separation of Variables, Stäckel Determinant
References
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part 1. New York: McGraw-Hill, p. 510, 1953.